Semiconjugacies between the Julia sets of
geometrically finite rational maps (Continued)

Tomoki Kawahira

Let us keep considering the family of polynomials parameterized by a complex number C,

F(z,C):=z(1+z)(1+z)(C-(2C+1)z+(3+4C)zz/4).

Recall that F(z,C) has the following properties:

1. z=0 is a fixed point with multiplier C.
2. z=-1 is a critical point and F(-1,C)=0.
3. z=1 is another critical point, and F(1,C)=-1, thus F(F(1,C),C)=0.

Since the map is degree 5, there are 2 more critical points other than 1 and -1. They are parameterize by C, like c1(C) and c2(C). Though it is not difficult to find the explicit formulae for c1(C) and c2(C), we restrict our attention to the perturbation of F(z,1), into another F(z, C) with C very close to 1. Now we have the following expansion about C=1:

c1(C)= 0.24257 + 0.11302 (C - 1) - 0.061795 (C - 1)^2 +...
c2(C)= -0.47114 + 0.017594 (C - 1) - 0.01284 (C - 1)^2 +...

Then c1(1) is the one attracted by the parabolic point z=0, and c2(0) is the other one attracted to the attracting point z=-0.30888... If the fixed point z=-0.30888... is perturbed then it still is attracting, and attracts c2(C). Thus bifurcation of F(z, 1) should be viewed by the critical orbit of c1(C).

Here are pictures of parameter plane C with respect to the orbit of c1(C). Actually I only used the linear part of the expansion above, but this nicely approximates the original near C=1.

Near C=1 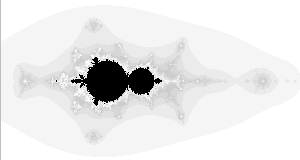Whole (approximated)

C=1 is the point where two black needles stick between the left and right black regions. f1 and f3 comes from the region on the right, and f2 comes from the region on the left. Now you want to take the value C from the sticking needles, don't you?

Case 4: C = 1.012 + 0.118 i

Set f4(z):=F(z, 1.012 + 0.118 i). Then the Julia set of f4(z) is as following:



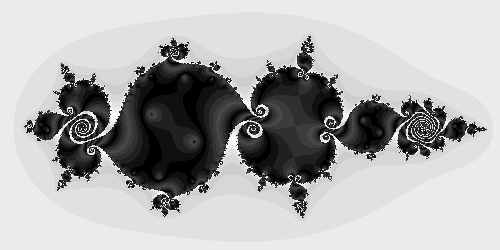
In this case, z=0 is perturbed into two repelling fixed points. Then both c1(C) and c2(C) are attracted to an attracting fixed point.

J(f4) near z=0, with the orbit of c1(C). J(f4) near z=1

Now the topology of the Julia set is completely changed. In this case, the theorem of the paper does not work. However, there exists a semiconjugacy between f3 and f0 on the Julia sets which comes from the dynamics of external rays.

Parameter plane colored by c2(C)

Next we see the parameter plane by using c2(C). Here we also use linear approximation.

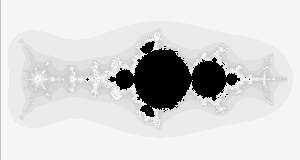Near C=1 Whole (approximated)

C=1 (the center of the figure on the left) is stable with respect to c2(C). However, another bifurcation occurs near C=0.920061.

First recall the picture of f1(z)=F(z, 1.05) by using c2(C). Now the gray region is the basin containing c1(C), and the region in gradation from black to dark gray is the basin containing c2(C).



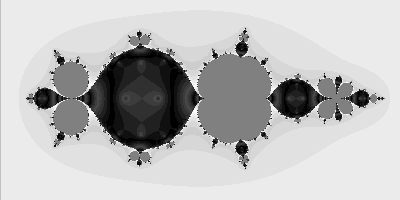
Second, this is the case of C=0.920.., new parabolic point appears at z=-0.160... Red points are the critical orbit of c2(C).



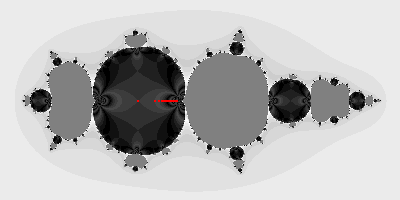
Third, C=0.91. The parabolic point splits into two repelling fixed points.



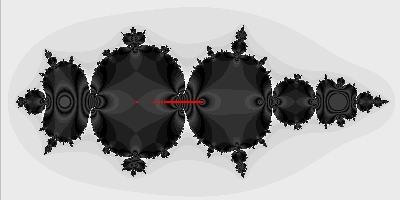
Forth, I lost the exact value but it was taken from the upper valley between two black regions near C=0.92 in the parameter picture. Critical orbit escapes to infinity. The region in gray is the attracting basin of z=0. which is now an attracting fixed point.



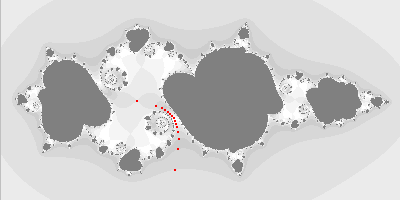
Fifth, I also lost the value. The picture on the right is parameter plane near this C.

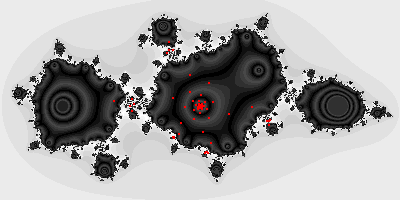
Many islands.

Appendix

Here are some other parameters, I lost the actual value of C, though..





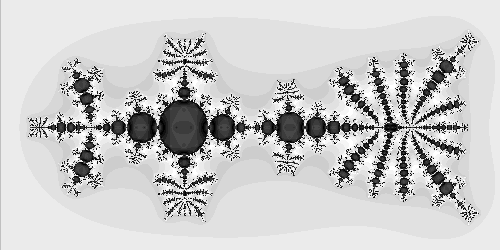
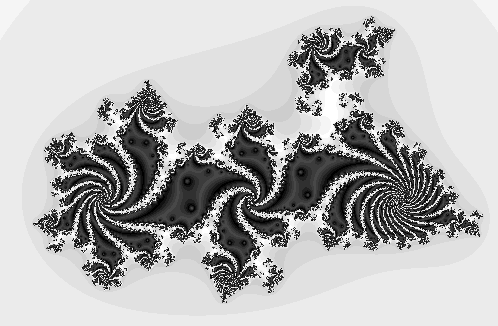
All pictures are generated by this program (tentatively I call it "OTIS-M" or "OTISM"). Enjoy "Tubes".