Semiconjugacies between the Julia sets of
geometrically finite rational maps

Tomoki Kawahira

A rational map is called geometrically finite if every critical point in the Julia set is eventually periodic. In my paper "Semiconjugacies between the Julia sets of geometrically finite rational maps" (in Erg. Th. and Dyn. Sys. 23(2003)), the following is shown: For suitable perturbations of a geometrically finite rational map, there exist conjugacies or "alomost injective" semiconjugacies between the dynamics on the perturbed Julia sets and the original one.

Here is an example in polynomial case: First, let us consider a geometrically finite polynomial,

f(z)=z(1+z)(1+z)(1-3z+1.75zz).

This polynomial has the following properties:

1. z=0 is a parabolic fixed point with one petal.
2. z=-1 is one of two critical points in the Julia set, and f(-1)=0.
3. z=1 is another critical point in the Julia set, and f(1)=-1, thus f(f(1))=0.
4. z=-0.30888.. is an attracting fixed point.

The following picture shows the filled Julia set of f:



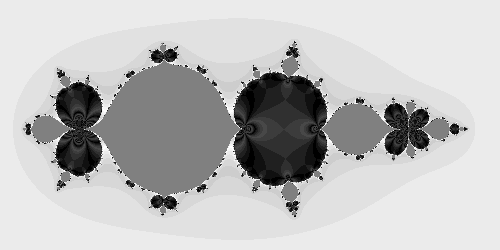
The region outside (colored in gradation from white to light gray) is the immediate basin of infinity. The gray region is the attracting basin of z=-0.30888... The region with cauliflower-like part (colored in gradation from black to dark gray) is the parabolic basin of z=0. The Julia set J(f) of f is the boundary between these three regions.

Next, let us consider the family of polynomials parameterized by a complex number C,

F(z,C):=z(1+z)(1+z)(C-(2C+1)z+(3+4C)zz/4).

Then for any C, F(z,C) has the following properties:

1. z=0 is a fixed point with multiplier C.
2. z=-1 is a critical point and F(-1,C)=0.
3. z=1 is another critical point, and F(1,C)=-1, thus F(F(1,C),C)=0.

Thus f(z) is the special case of C=1, that is,

f(z)=F(z,1).

So changing C of F(z,C) near C=1 gives a perturbation of f(z).

Case 1: C=1.05

Set f1(z):=F(z,1.05). Then the Julia set of f1(z) is as following:



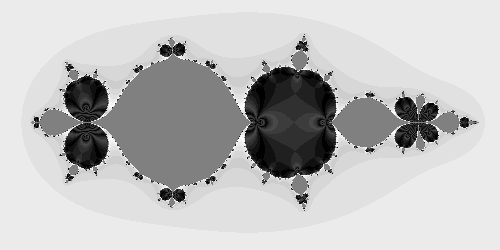
In this case, z=0 is perturbed into a repelling fixed point with multiplier 1.05. The original parabolic point splits into a pair of repelling and attracting fixed points. Here are the pictures near 0, centered at 0, and with critical orbits drawn in.

J(f) near z=0 (original) J(f1) near z=0 (perturbed)

However, the topology ("shape") of the Julia set is not changed. By the main theorem in the paper, there exists a topological conjugacy h1: J(f1) -> J(f); that is, the dynamics on the both Julia sets are essentially the same.

Case 2: C=0.95

Set f2(z):=F(z,0.95). Then the Julia set of f2(z) is as following:



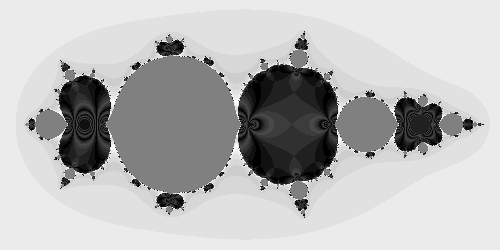
In this case, z=0 is perturbed into an attracting fixed point with multiplier 0.95. The original parabolic point again splits into a pair of repelling and attracting fixed points.

J(f2) near z=0, centered at 0.
Now the topology of the Julia set is slightly changed. Indeed, J(f) and J(f1) contain z=-1 and z=1 (critical points) but J(f2) does not. z=-1 and z=1 land on z=0, which is now perturbed into an attracting point.

However, by the main theorem in the paper, there exists a semiconjugacy h2: J(f2) -> J(f). Moreover, except at the preimages (backward orbits) of z=0 of f, h2 gives a topological conjugacy. That is, the dynamics on the both Julia sets are "almost" the same.

Case 3: C=1.02+0.05i

Set f3(z):=F(z,1.02+0.05i). Then the Julia set of f3(z) is as following:



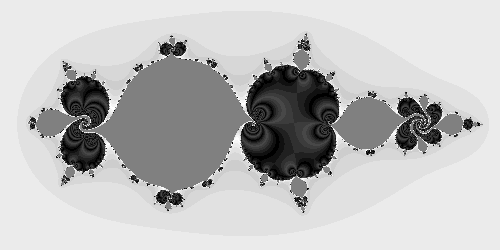
In this case, as in Case 1, z=0 is perturbed into a repelling fixed point (with multiplier 1.02+0.05i). The original parabolic point also splits into a pair of repelling and attracting fixed points. Note that the attractive one is not on the real axis.

J(f3) near z=0, and a critical orbit.

Though the original parabolic basin is changed to be swirling near z=-1, 0, 1 and so on, the topology of the Julia set is not changed as in Case 1.

J(f3) near z=1

Actually, there exists a topological conjugacy h3: J(f3) -> J(f) by the theorem.

For more examples, see Tiles 2 .

Next let us go to the parameter planes.